Problem of the Week #80: Monday February 10th, 2025
As before, these problems are the results of me following my curiosity, and I make no promises regarding the topics, difficulty, solvability of these problems.
Please register for an account if you would like to join the discussion below or share your own problems.
This problem is inspired by the 1973 USAMO Q2.
A Lucas Sequence is made from two starting terms and a recursive formula for the next term using the two previous ones, like on the left.
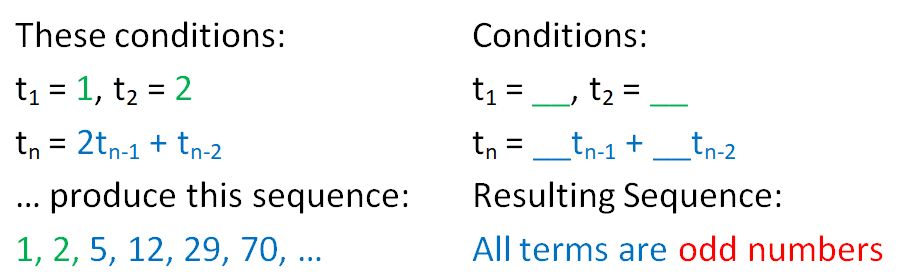
a). Construct a Lucas Sequence that contains only odd numbers.
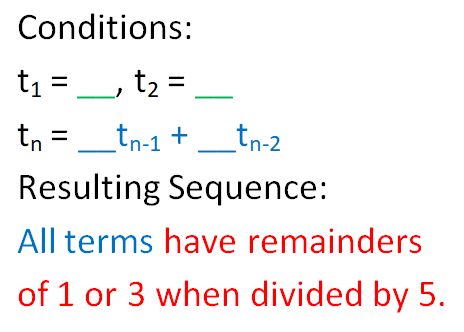
b). Construct a Lucas Sequence that contains only numbers that give a remainder of 1 or 3 (infinitely many of each) when divided by 5.
c). Construct a Lucas Sequence that has no number in common with the sequence from the previous question.

d). Construct a Lucas Sequence that has no number in common with the Fibonacci Sequence.

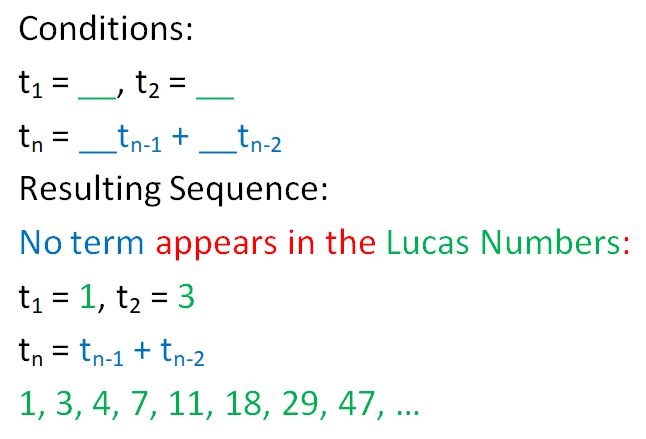
e). Construct a Lucas Sequence that has no number in common with the Lucas Numbers.
f). Is it possible to construct a Lucas Sequence that has numbers with all possible remainders when divided by any finite positive integer?

g). Share your own problem inspired by this one.

h). Give one of these questions to a friend/colleague/student/family member to start a mathematical discussion.
